3.8: Jacobians
- Page ID
- 582
Consider the integral
\[ \int _0^2 x\, \text{cos}\, (x^2) \; dx. \]
To evaluate this integral we use the u-substitution
\[ u = x^2. \]
This substitution sends the interval \([0,2]\) onto the interval \([0,4]\). We can see that there is stretching of the interval. The stretching is not uniform. In fact, the first part \([0,0.5]\) is actually contracted. This is the reason why we need to find \(du\).
\[ \dfrac{du}{dx} = 2x \]
or
\[ \dfrac{dx}{du} = \dfrac{1}{2x}. \]
This is the factor that needs to be multiplied in when we perform the substitution. Notice for small positive values of \(x\), this factor is greater than 1 and for large values of \(x\), the factor is smaller than 1. This is how the stretching and contracting is accounted for.
We have seen that when we convert 2D Cartesian coordinates to Polar coordinates, we use
\[ dy\,dx = r\,dr\,d\theta \label{polar}\]
with a geometrical argument, we showed why the "extra \(r\)" is included. Taking the analogy from the one variable case, the transformation to polar coordinates produces stretching and contracting. The "extra \(r\)" takes care of this stretching and contracting. The goal for this section is to be able to find the "extra factor" for a more general transformation. We call this "extra factor" the Jacobian of the transformation. We can find it by taking the determinant of the two by two matrix of partial derivatives.
Definition: Jacobian for Planar Transformations
Let
\[ x = g(u,v) \]
and
\[y = h(u,v) \]
be a transformation of the plane. Then the Jacobian of this transformation is
\[ \begin{align} \dfrac{\partial(x,y)}{\partial(u,v)} &= \begin{vmatrix} \dfrac{\partial x}{\partial u} & \dfrac{\partial x}{\partial v} \nonumber \\[4pt] \dfrac{\partial y}{\partial u} & \dfrac{\partial y}{\partial v} \end{vmatrix} \\[4pt] &= \dfrac{\partial x}{\partial u} \dfrac{\partial y}{\partial v} - \dfrac{\partial y}{\partial u} \dfrac{\partial x}{\partial v}. \label{Jacob2D} \end{align} \]
Example \(\PageIndex{1}\): Polar Transformation
Find the Jacobian of the polar coordinates transformation \( x(r,\theta) = r \cos \theta \nonumber \) and \( y(r,q) = r \sin \theta . \nonumber\).
Solution
This is a direct application of Equation \ref{Jacob2D}. We have
\[ \begin{align*} \dfrac{\partial (x,y)}{\partial (r,\theta)} &= \begin{vmatrix} \cos \theta & -r\sin \theta \nonumber \sin \theta & r \cos \theta \end{vmatrix} \\[4pt] &= r\cos^2 \theta + r\sin^2 \theta \\[4pt] &= r \end{align*}.\]
This is comforting since it agrees with the extra factor in integration (Equation \ref{polar}).
2D Jacobians
Theorem: Integration and Coordinate Transformations
Let
\[T: \mathbb{R}^2 \rightarrow \mathbb{R}^2 \]
given by
\[ x = g(u,v) \;\;\; \text{and} \;\;\; y = h(u,v)\]
be a transformation on the plane that is one to one from a region \(S\) to a region \(R\). If \(g\) and \(h\) have continuous partial derivatives such that the Jacobian is never zero, then
\[\iint\limits_{R} f(x,y) \; dy\,dx = \iint f(g(u,v),h(u,v))\left|\dfrac{\partial(x,y)}{\partial(u,v)}\right|\; du\,dv.\]
Remark: A useful fact is that the Jacobian of the inverse transformation is the reciprocal of the Jacobian of the original transformation.
\[ \dfrac{\partial (x,y)}{\partial(u,v)} = \dfrac{1}{\left|\dfrac{\partial(u,v)}{\partial (x,y)}\right|}\]
This is a consequence of the fact that the determinant of the inverse of a matrix \(A\) is the reciprocal of the determinant of \(A\).
Proof
As usual, we cut \(S\) up into tiny rectangles so that the image under \(T\) of each rectangle is a parallelogram.
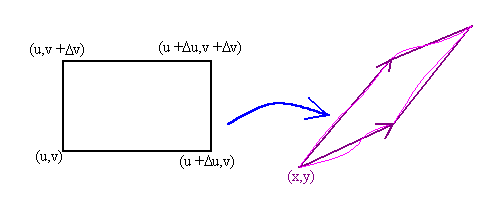
We need to find the area of the parallelogram. Considering differentials, we have
\[ T(u + \Delta u,v) = T(u,v) + (x_u\Delta u,y_u\Delta u) \nonumber\]
\[ T(u,v + \Delta v) = T(u,v) + (x_v\Delta v,y_v\Delta v). \nonumber\]
Thus the two vectors that make the parallelogram are
\[ \begin{align*} \vec{P} &= x_u \Delta u \hat{\textbf{i}} + y_u \Delta u \hat{\textbf{j}} \\[4pt] \vec{Q} &= x_v \Delta v \hat{\textbf{i}} + y_v \Delta v \hat{\textbf{j}}. \end{align*}\]
To find the area of this parallelogram we just cross the two vectors.
\[ \begin{align*} P \times Q &= \begin{vmatrix} \hat{\textbf{i}} &\hat{\textbf{j}} &\hat{\textbf{k}} \nonumber \\[4pt] x_u \Delta u &y_u \Delta u &0 \nonumber \\[4pt] x_v \Delta v & y_u \Delta v &0 \end{vmatrix} \\[4pt] &= \left|x_u y_v - x_v y_u \right| \Delta u \Delta v \\[4pt] &= \left| \dfrac{\partial (x,y)}{\partial(x,y)} \right| \Delta u\, \Delta v \end{align*} \]
and the extra factor is revealed.
\(\square\)
Example \(\PageIndex{2}\)
Use an appropriate change of variables to find the volume of the region below
\[ z = (x - y)^2 \nonumber\]
above the x-axis, over the parallelogram with vertices \((0,0)\), \((1,1)\), \((2,0)\), and \((1,-1)\).

Solution
We find the equations of the four lines that make the parallelogram to be
\[y = x \;\;\; y = x - 2 \;\;\; y = -x \;\;\; y = -x + 2\nonumber\]
or
\[ x - y = 0 \;\;\; x - y = 2 \;\;\; x + y = 0 \;\;\; x + y = 2.\nonumber\]
The region is given by
\[0<x-y<2 \;\;\; \text{and} \;\;\; 0<x+y<2 .\nonumber\]
This leads us to the inverse transformation
\[u(x,y) = x - y \;\;\; v(x,y) = x + y.\nonumber\]
The Jacobian of the inverse transformation is
\[\dfrac{\partial (u,v)}{\partial (x,y)} = \begin{vmatrix} 1 &-1 \nonumber \\[4pt] 1 &1 \end{vmatrix} =2. \nonumber\]
Since the Jacobian is the reciprocal of the inverse Jacobian we get
\[ \dfrac{\partial (u,v)}{\partial (x,y)} = \dfrac{1}{2}.\nonumber\]
The region is given by
\[ 0 < u < 2 \;\;\; \text{and} \;\;\; 0 < v < 2 \nonumber\]
and the function is given by
\[ z = u^2. \nonumber\]
Putting this all together, we get the double integral
\[\begin{align*} \int_0^2 \int_0^2 u^2 (\dfrac{1}{2})\; dudv &= \int_0^2 \left[ \dfrac{u^3}{6} \right]^2 \; dv \nonumber \\[4pt] &= \int_0^2 \dfrac{4}{3}\; dv \nonumber \\[4pt] &= \dfrac{8}{3}. \end{align*}\]
3D Jacobians
For transformations from \(\mathbb{R}^3\) to \(\mathbb{R}^3\), we define the Jacobian in a similar way
\[ \dfrac{\partial (x,y,z)}{\partial (u,v,w)} = \begin{vmatrix} x_u & x_v & x_w \\[4pt] y_u & y_v & y_w \nonumber \\[4pt] z_u & z_v & z_w \end{vmatrix}. \label{Jacob3D} \]
Example \(\PageIndex{3}\): Spherical Transformation
Find the Jacobian for the spherical coordinate transformation
\[ x = r\, \cos\,\theta \; \sin\,\phi \;\;\;\;\; y = r\, \sin\, \theta\; \sin\, \phi \;\;\;\;\; z = r\, \cos\, \phi. \nonumber\]
Solution
We take partial derivatives and compute
\[\begin{align*} \dfrac{\partial (x,y,z)}{\partial (\rho, \theta, \phi)} &= \begin{vmatrix} \cos \theta \sin \phi & -\rho \sin \theta \sin \phi & \rho \cos \theta \cos \phi \nonumber \\[4pt] \sin \theta \sin \phi & \rho \cos \theta \sin \phi & \rho \sin \theta \cos \phi \nonumber \\[4pt] \cos \phi & 0 & -\rho \sin \phi \end{vmatrix} \nonumber \\[4pt] &= | \cos \theta \sin \phi (-\rho^2\cos \theta \sin^2 \phi) + \nonumber \\[4pt]& \;\;\; \rho \sin\theta \sin\phi (-\rho \sin\theta \sin^2\phi - \rho \sin\theta \cos^2\phi) + \rho\cos\theta \cos\phi(-\rho \cos\theta \cos\phi \sin\phi) \nonumber \\[4pt] &= -\rho^2 \cos^2\theta \sin^3\phi - \rho^2 \sin^2\theta \sin\phi (\sin^2\phi + \cos^2\phi) - \rho^2\cos^2\theta \cos^2\phi \sin\phi \nonumber \\[4pt] &= \rho^2 \sin\phi(\cos^2\theta\sin^2\phi + \sin^2 \theta + \cos^2\theta\cos^2\phi) \nonumber \\[4pt] &= \rho^2 \sin\phi (\cos^2\theta (\sin^2\phi + \cos^2\phi) + \sin^2\theta) \nonumber \\[4pt] &= \rho^2 \sin\phi (\cos^2\theta + \sin^2\theta) \nonumber \\[4pt] &= \rho^2 \sin\phi .\end{align*} \]
Contributors and Attributions
- Larry Green (Lake Tahoe Community College)
Integrated by Justin Marshall.


